Cartographer建图问题
本文共 500 字,大约阅读时间需要 1 分钟。
目前手上只有个16线的激光雷达,想在房间跑一下建图,目的是能够跑通就可以.
1,启动激光雷达, 2,将激光雷达3d点云转成2d laserscan数据类型 3,启动cartographer包roslaunch cartographer_ros agv_2d.launchCartographer配置:
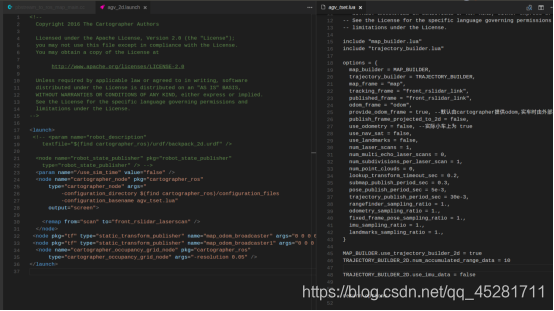
终端报错:
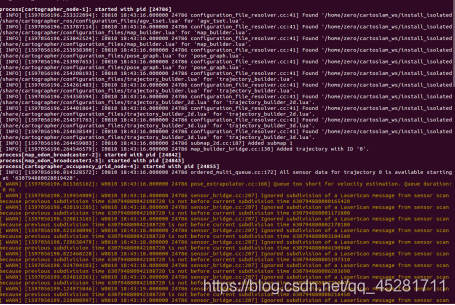
百度谷歌了一大圈:
都说是 的问题,我把它设置成false或者注释掉都不好使.大佬可有遇到过这种情况吗?解决:
激光雷达里面设置的仿真时间,去掉之后就好<arg name="time_synchronization" default="false" /> 来cartographer launch里面这个要打开,这是显示map的节点!!!
<node name="cartographer_occupancy_grid_node" pkg="cartographer_ros" type="cartographer_occupancy_grid_node" args="-resolution 0.05" /> cartographer包中的任何文件改动后均需要重新编译运行!!!
转载地址:http://fmntz.baihongyu.com/
你可能感兴趣的文章
Mysql学习总结(38)——21条MySql性能优化经验
查看>>
Mysql学习总结(45)——Mysql视图和事务
查看>>
Mysql学习总结(58)——深入理解Mysql的四种隔离级别
查看>>
Mysql学习总结(59)——数据库分库分表策略总结
查看>>
Mysql学习总结(80)——统计数据库的总记录数和库中各个表的数据量
查看>>
Mysql学习总结(83)——常用的几种分布式锁:ZK分布式锁、Redis分布式锁、数据库分布式锁、基于JDK的分布式锁方案对比总结
查看>>
MySQL定义和变量赋值
查看>>
mysql实现成绩排名
查看>>
Mysql客户端中文乱码问题解决
查看>>
mysql导入数据库出现:Incorrect string value: '\xE7\x82\xB9\xE9\x92\x9F' for column 'chinese' at row 1...
查看>>
Mysql工作笔记006---Mysql服务器磁盘爆满了_java.sql.SQLException: Error writing file ‘tmp/MYfXO41p‘
查看>>
MySQL底层概述—5.InnoDB参数优化
查看>>
MySQL底层概述—6.索引原理
查看>>
Mysql建立中英文全文索引(mysql5.7以上)
查看>>
Mysql当前列的值等于上一行的值累加前一列的值
查看>>
MySQL当查询的时候有多个结果,但需要返回一条的情况用GROUP_CONCAT拼接
查看>>
MySQL必知必会(组合Where子句,Not和In操作符)
查看>>
MySQL必知必会总结笔记
查看>>
MySQL快速入门——库的操作
查看>>
mysql快速复制一张表的内容,并添加新内容到另一张表中
查看>>